HIGHLIGHTS
• The Archard wear equation provides a simple approach for predicting wear when two surfaces are in a steady-state but not during the transient running-in period.
• An extension of the Archard wear equation is now reported that uses a logistic function originally developed to model population growth to accurately describe bearing ratio curves during the running-in period. 
• A custom ring-on block tribometer was used to verify the extended Archard wear equation. 

Minimization of wear remains a long-standing objective for tribologists particularly as end-use applications are conducted under more stressful operating conditions over longer periods of time. Predicting wear, or the removal of material at the points that two surfaces interact with each other during the operation of machinery, has been done using the Archard wear equation.

STLE member Dr. Michael Varenberg, senior research engineer for John Crane Inc. in Morton Grove, Ill., says, “The Archard wear equation provides a simple approach for predicting wear by suggesting worn volume to be directly proportional to the normal load and sliding distance and inversely proportional to the hardness of the softer surface. A parameter that defines the proportionality is the dimensionless wear coefficient.”

Varenberg provided an example of how the Archard wear equation can help in practice. He says, “Tools used in material removal operations, such as cutting, have to meet tight tolerances when machining work pieces. If these tolerances are not met, then the parts produced may not be functional. The Archard wear equation can be used to predict how a specific cutting tool will perform in a particular operation.”

Another application using the Archard wear equation was discussed in a previous TLT article¹ that dealt with the wear rate of monetary coins. With the cost of metals rising, efforts have been underway to find the right alloys to be used in coins so they can remain in circulation for an extended period of time. The Archard wear equation was used based on the assumption that coins are worn by rubbing rather than corrosion. A modification of the Archard wear equation was developed to take into account that the material loss occurs over a specific time frame which is introduced into the equation.

Coins consist of two-level relief areas where the high regions wear faster than the low regions. The Archard wear equation demonstrates that harder coins will last longer because they will not wear as readily during use.

One weakness of the Archard wear equation is that it does not account for wear during the transient running-in period as a specific machine starts to operate. Varenberg says, “The Archard wear equation is very effective in predicting wear when two surfaces are in a steady-state rubbing, after the running-in period is over. But the challenge with predicting wear during the running-in is due to the changing contact area between surfaces that gradually adapt to specific loading conditions. The process starts with rough surfaces contacting each other at the various tips of highest asperities that are quickly worn as other contact points are established on the surfaces. Pressure at the tips of these asperities is quite high meaning the wear rate is more extensive than what occurs during the steady-state regime when the contact area remains constant.”

Past work to determine how to predict wear during the running-in period has pointed to the importance of surface roughness. Varenberg says, “Older research that correlated roughness to running-in dates back to before computers were routinely used in science, so regular surface texture was employed to simplify analysis. Later, assumptions were made suggesting that rough surfaces can be represented by average asperities of specific radius and density having typical Gaussian height distribution. The problem is that these assumptions were not always correct, leading to inaccurate results.”

Varenberg now reports that the Archard wear equation can be extended to account for wear occurring during the running-in period.

Logistic function
The challenge facing Varenberg was to define the change in contact area as the original surface is removed from 0% to 100%, during running-in. The solution was in employing the material ratio curve.

Varenberg says, “Bearing ratio curves that present surface material as a function of height can be used to explain how wear changes during the running-in period. These empirical data can be plugged in directly but to simplify the process, the logistic function, originally derived to model population growth, can be deployed. The logistic function is well suited to accurately describing bearing ratio curves of the original unworn surface.”

The logistics function was then calibrated against measured bearing ratio curves. This enabled Varenberg to extend the Archard wear equation to account for wear during the running-in period.

A custom ring-on block tribometer was then used to verify the extended Archard wear equation (see Figure 3). Varenberg says, “The interaction of two materials (ring sample made from mild steel SAE 1045 and block samples prepared from polytetrafluoroethylene) that exhibit a relatively high wear ratio and low stable friction was used in the evaluation process. This allowed for determining the validity of the wear equation in a short period of time.”


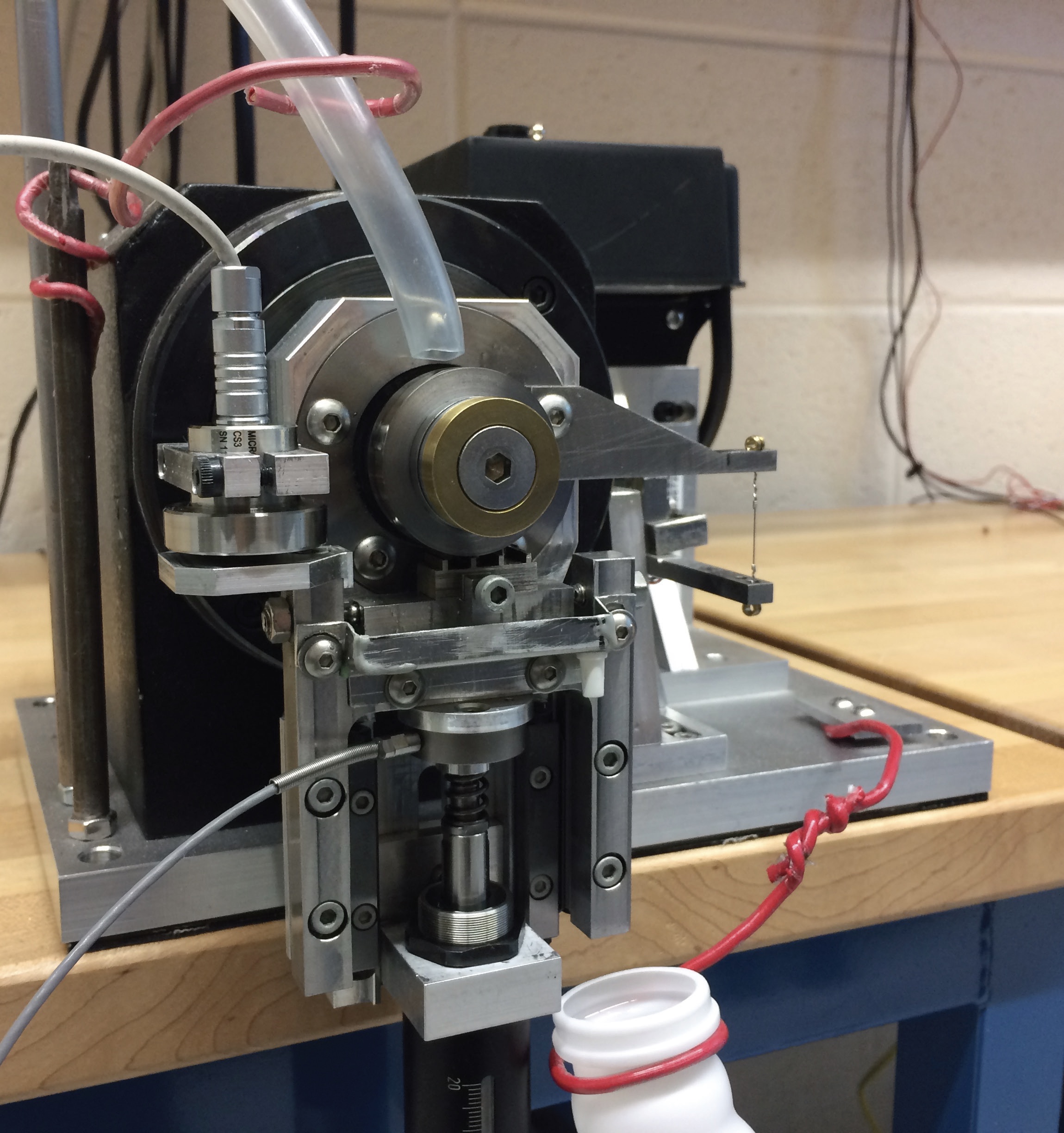Figure 3. A custom ring-on block tribometer was used to verify that the Archard Wear equation can be extended to the transient running-in period. Figure courtesy of John Crane Inc.

The ring sample is fixed to the spindle’s shaft and rotated at a constant speed. The block sample contains a protruding tooth that comes into contact with the ring under a constant normal load. Wear depth is measured by the change in relative position between the ring and the block. Experiments were conducted until the tooth was completely worn.

Varenberg says, “The difficulty in doing these experiments was the need to roughen the protruded tooth with sandpaper before each test and then measure the surface roughness using a portable profilometer without disassembling the block sample from the rig.”

The results from the wear experiments confirmed the accuracy of the extended Archard wear equation. Varenberg says, “This extension makes the Archard wear equation a much more powerful tool for predicting wear during the interaction of two surfaces not just during steady-state running but also when two surfaces adapt to contact with each other. It is anticipated that this approach will be instrumental in applications where thin coatings are applied. There also is no doubt that the use of the logistic description of rough surfaces will become helpful in other contact problems.”

Additional information can be found in a recent article² or by contacting Varenberg at michael.varenberg@johncrane.com.

REFERENCES
1. Tysoe, W. and Spencer, N. (2017), “A thought for your pennies,” TLT, 73 (4), p. 80. Available here.
2. Varenberg, M. (2022), “Adjusting for running-in: Extension of the Archard wear equation,” Tribology Letters, Article Number: 59.