A universal model for the static friction coefficient in a full stick elastic-plastic coated spherical contact

Chen Z. and Etsion I.

Dept. of Mechanical Engineering, Technion, Haifa, 32000, Israel

INTRODUCTION: For decades, the coating technology has been widely used in industry to control the surface friction property. However, the selection of some important parameters in coating application such as coating thickness and coating material still mainly relies on empiricism [1].

It is reasonable to assume that the contact between coated surfaces is localized to many individual coated spherical tips (see e.g. the classic GW model [2]). Research concerning coated spherical contact under combined normal and tangential loading is relatively meager and limited to elastic regime [3] and those on elastic-plastic regime were mostly done for a homogeneous sphere [4]. Brizmer et. al. [4] found in stick condition that the increasing tangential load leads to a decreasing tangential stiffness that finally vanishes at sliding inception.

The aim of the present paper is to investigate the elastic-plastic coated spherical contact under combined normal and tangential loading in full stick contact condition.

FINITE ELEMENT MODEL:  A coated spherical contact under combined normal and tangential loading is schematically presented in Fig. 1, where the coated sphere is composed of a substrate of radius R and a coating of thickness t. To solve this complex elastic-plastic contact problem, the finite element method, executed by the commercial software ANSYS 18.1, was used. A constant normal load P was first applied to the rigid flat. Subsequently, a stepwise increased tangential displacement (u_x)_i was applied to the rigid flat, where i is the consecutive number of the tangential displacement step. The tangential force Q_i can be obtained as the sum of the x component of reaction of nodes at sphere bottom. The tangential stiffness (K_T)_i is thus (Q_i – Q_i-1)/((u_x)_i - (u_x)_i-1). The criterion for sliding inception in Ref. [4] was also used here, i.e., sliding incepts once (K_T)_i ≤ 0.1(K_T)₁.   

Figure 1 A coated sphere under combined normal and tangential loading.

RESULTS AND DISCUSSION:  Fig. 2 presents the static friction coefficient m as a function of t/R when E_co/E_su = 4, E_co/Y_co = E_su/Y_su = 1000 under the dimensionless normal load P^* = 50, where E and Y indicate Young’s modulus and yield strength, respectively, and subscripts ‘co’ and ‘su’ indicate coating and substrate material, respectively. P^* is defined as P/(E_suR²×10^-7). Since E_su and R are fixed, the dimensional normal load P is proportional to P^*. Thus, results in Fig. 2 are in fact obtained under the same dimensional normal load P. As a result, only the effect of the geometry parameter t/R on the static friction coefficient is revealed in Fig. 2. The static friction coefficient m first increases linearly with t/R from m_su at t/R = 0 till reaching a maximum m_m at (t/R)_m. Further increase of t/R above (t/R)_m leads to a decreasing m that eventually approaches m_co.

In a homogeneous spherical contact [4], a more plastic and compliant junction results in a lower m and a junction of larger size can support a larger friction force. It was also found that a thicker hard coating better protects the substrate from plastic deformation and results in a smaller contact area at the coating outer surface [5]. Hence, for t/R from 0 to (t/R)_m, where the substrate is the dominant component of the coated system, increasing t/R will reduce the plasticity level in the substrate and therefore increase m. On the other hand for t/R above (t/R)_m where the coating is the dominant component increasing t/R will reduce the contact area thereby reducing m.

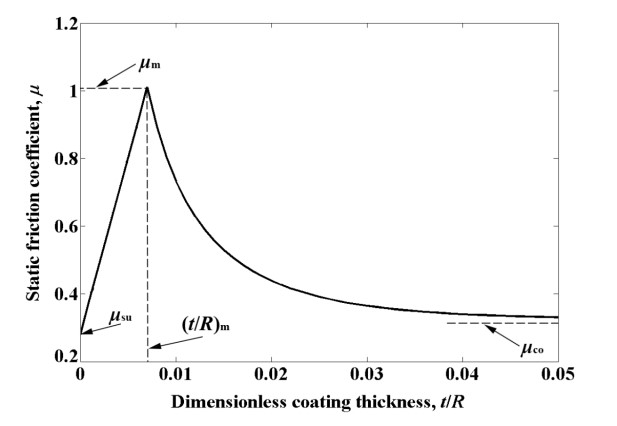
Figure 2 The static friction coefficient m as a function of t/R for E_co/E_su = 4, E_co/Y_co = E_su/Y_su =1000 and P^* = 50.

REFERENCES:  1. Holmberg, Surf. Coat. Tech. (2007), 2. Greenwood, T. Roy. Soc. (1966), 3. Keer, Int. J. Solids. Struct. (1991), 4. Brizmer, Tribo. Let.t (2007), 5. Chen, Tribo. Int.      (2017).